1.3 VOLUMES OF GASES, IDEAL GAS EQUATION & THE MASS SPECTROMETER

IF YOU WOULD LIKE A COPY OF PAST PAPER QUESTIONS FOR THIS TOPIC OR ANY OTHER TOPIC PLEASE EMAIL ME (space is provided on the main page of my blog)

IF YOU WOULD LIKE TO GO THROUGH THE QUESTIONS WITH ME, PLEASE VISIT MY YOUTUBE CHANNEL

Hi everyone! It's Chemistry at glance. Today is the last post on chemical calculations. And with that another youtube video with past paper questions. There is a lot to take in for this unit and I also know that there are couple of equations you need to remember. However, practice makes it perfect. The more examples you do, the faster you will remember these equations.

Also, the questions on chemical calculations are quite repetetive, therefore past paper questions are the way to go!

Volume of gases:

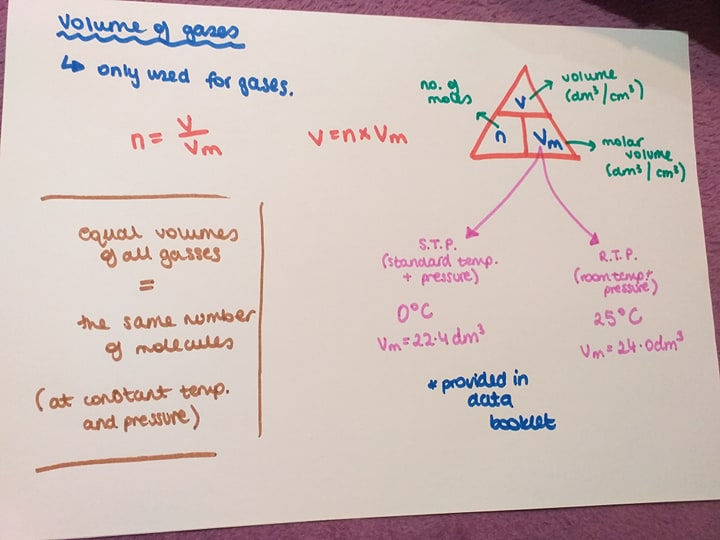
Number of moles (n)= volume (v)/ molar volume (Vm)

This equation is only used for gases. Molar volume is going to be one of the two: 22.4dm^3 or 24dm^3. One is for a standard temperature and pressure and the other is room temperature and pressure. You know which one to use because they will hint this in the question. They will either say standard or room temp. or they will give you the temp. 0 degrees Celcius (standard) or 25 degrees Celcius (room). Of course this is provided in the data booklet.

If you have two gasses and they both have the same volume, this means they both have the same number of molecules. This is what Avogadro stated.

And now I have an example:

- Like I told you, they are hinting in the question what molar mass you need to use. They are saying 'at room temperature and pressure' therefore it is 24dm^3.

- Next, thing you should realise is that they are giving you mass for another molecule, so you need to use this molecule to find the number of moles of carbon dioxide (molecules you are trying to find the volume for).

- Let's start with calculations then, n=m/Mr for calcium carbonate. The ratio is 1:1 with carbon dioxide, so the number of moles is exactly the same as for calcium carbonate. Lastly, use n=v/Vm and rearrange to calculate volume.

---

The ideal gas equation:

There are three laws that form the ideal gas eqution.

1. Boyle's law - his law states that at fixed temperature, the volume of gas is inversely proportional to pressure. V=1/p

2. Charle's law - his law states that at constant pressure the volume of gas is proportional o temperature. V=T

3. Avogadro's law - he states that equal volumes of gases have the same number of molecules. V=n

Combining all of these equations (it iis quite easy because they are all equal to volume) you get V=nT/p. This is nearly our ideal gas equation but what you are missing is a gas constant and this is represented as R. Therefore what you get is V=nTR/p.

The equation is usually represented in a rearranged form which is pV=nRT.

p - pressure, measured in Pascals. You alway need to change the pressure to Pascals. Data booklet may help you in doing just so.

V - volume, measured in m^3. Always change from dm^3 (x10^-3) and from cm^3 (x10^-6) to m^3, otherwise the equation will not work. Again data booklet helps in doing so.

n - number of moles of gas, measured in mol. This number you might be asked to calculate first using a different equation.

R - gas constant, which is 8.314, you will be given this in the question.

T - temperature, measured in Kelvins. If you are given a temperature in degrees Celcius, be sure to conert it to Kelvins. In irder to do this you do 273+degrees Celcius.

examples...

e.g.(1)

In this type of question I usually just write down all of the values that are needed for the equation and convert to appropriate units.

Then, rearrange the equation depending on what you are trying to find.

e.g.(2)

This question is a little bit more complicated. You are told to calculate the Mr of the compound X. You are privded with the mass however we don't have the number of moles 'n' in order to use the equation n=m/Mr.

But, we have pressure, temperature, volume and the gas constant (convert it to appripriate units), so you can find the number of moles with no problem. Of course remember to rearrange the equation.

Knowing the number of moles, you can now use n=m/Mr to find the Mr of compound X.

e.g.(3)

The ideal gas equation can be used differently as well. If the number of moles and the gas constant stay the same throughout the entire question, you can just take it out of the equation.

This leaves us with pV/T. You have one set of numbers that fit into this equation and you are trying to find the volume of the second set of data.

You have all of the values, they just need converting into apprpriate units and putting into the equation. And here you are, you have the volume.

I think these 3 examples are all the ways they may ask you to use this ideal gas equation.

---

The mass spectrometer:

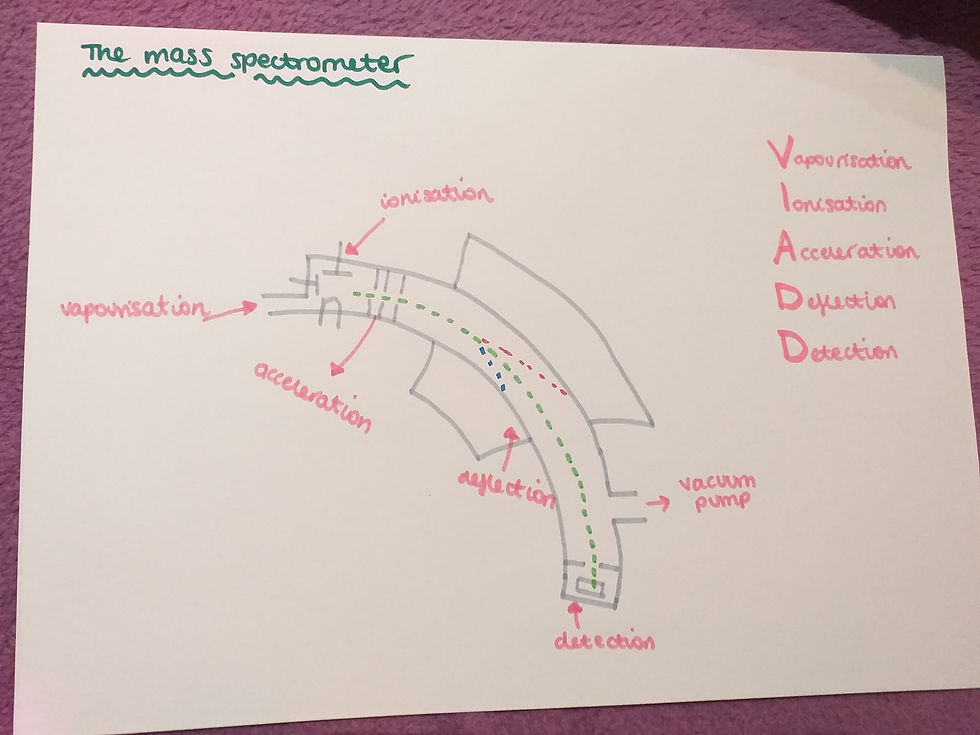
This is a mass spectrometer and you can see all of the stages that happen in a mass spectrometer on the image above. This is something you need to know. There is no need for you to be able to draw the mass spectrometer though.

These are the stages:

Vapourisation - before the sample is put into the mass spectrometer, you need to vapourise the sample.

Ionisation - in this stage atoms are bombarded with high energy electrons. This is because all atoms need to be positive ions to go further the mass spectrometer. Because the electrons have lot of energy they knock the electrons from the atoms, making them positive. And this is what we are aiming for.

Acceleration - all the positive ions are accelerated to have the same kinetic energy. This means they are all travelling with the same speed.

Deflection - in this stage positive ions are deflected by the magnetic field. The amount of this deflection depends on two things. First one is the mass: the lighter the ion, the more it is deflected.

The second factor is the charge: the more positive the ion, the bigger will be the deflection (more positive means it will have a +3 instead of +1 for example). Both of these factoras are combined to a mass/charge ratio, m/z.

Detection - in this part only the positive ions, which haven't been deflected, will be detected. All the other positive ions, which have been deflected, will hit the walls of the mass spectrometer, gain electrons and become neutralised. They will be removed by the vacuum pump (this is drawn on the picture with mass spectrometer).

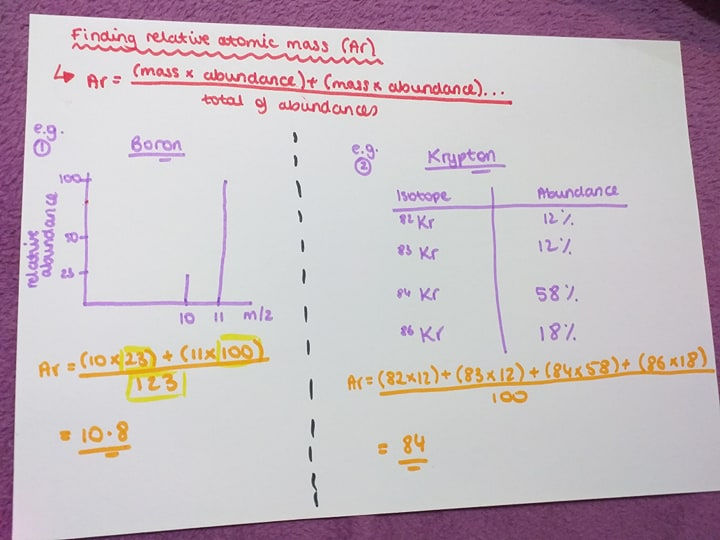
You can find the relative atomic mass, Ar, of an atom using the mass spectrometer. Here is an equation for this:

Ar= (mass x abundance) + (mass x abundance)/ total of abundances

You would either get a table with results or a graph showing m/z (mass) and relative abundance. I believe the rest is self- explanatory, just look at the image above and slowly go through it.

Afer you find the answer you can check if it is correct by looking at the periodic table. E.g. for the first example the Ar we calculated is 10.8 for Boron. Looking a the periodic table, the relative atomic mass for Boron is 10.8, therefore our answer is correct.

Chlorine mass spectrum:

This is an example of the results from a mass spectrometer for chlorine, put in a graph.

- Chlorine has two isotopes 35-Cl and 37-Cl.

- 35-Cl is three times more common than 37-Cl isotope, therefore the peak ratio in the graph is 3:1;

- The isotopes can also combine in a mass spectrometer and they can combine in different ways;

- All the possible combinations are visible in the image above and these combinations give a ratio of 9:6:1.

This mass spectrometer is an example of how a mass spectrometer should look like. In an exam you might be asked to draw a different graph for a different isotope (e.g. iodine) but based on chlorine graph. (You are welcomed to visit my youtube channel with past paper questions. I go through such an example there).

Fragmentation patterns:

This graph shows results from a mass spectrometer of a molecule of pentane. This graph shows all of the fragments, that pentane divides into, in a mass spectrometer. As we said before, all of the fragments must be positive because otherwise they would not be detected.

- The highest peak on this graph is called the 'Base peak'. This is the most common fragment of pentane.

- The peak with the highest m/z is called the 'Molecular ion peak' and this shows the Mr of the molecules, in this example the Mr of pentane.

- On the image above you will see different m/z numbers and the fragments that are responsible for this peak.

- The last thing you need to know is how to recognise these fragments. The m/z of these fragments is basically the Mr of this fragment. So, just count it to see what atoms will get into the fragment.

YOU ALWAYS NEED TO REMEMBER TO PUT A PLUS AND A GAS STATE NEXT TO THE FRAGMENT BECAUSE YOU MIGHT LOSE MARKS FOR THIS.

This is everything you nned to know for chemical calculation. See you next time!

PS. Please remember, I am only a student, and as anyone, I can make mistakes. If you think you can see one, don't hesitate and comment (either here on on my youtube channel) Thank you!